RESEARCH ARTICLE


Comparing Measures of Association in 2×2 Probability Tables



Dirk Hasenclever1, *, Markus Scholz1, 2
1 Institute for Medical Informatics, Statistics and Epidemiology, Leipzig, Germany
2 LIFE Research Center University of Leipzig, Leipzig, Germany

Article Information


Identifiers and Pagination:

Year: 2016
Volume: 7
First Page: 20
Last Page: 35
Publisher Id: TOSPJ-7-20
DOI: 10.2174/1876527001607010020

Article History:

Received Date: 23/03/2015
Revision Received Date: 25/04/2016
Acceptance Date: 02/05/2016
Electronic publication date: 23/08/2016
Collection year: 2016

© Hasenclever and Scholz; Licensee Bentham Open.

open-access license: This is an open access article licensed under the terms of the Creative Commons Attribution-Non-Commercial 4.0 International Public License (CC BY-NC 4.0) (https://creativecommons.org/licenses/by-nc/4.0/legalcode), which permits unrestricted, non-commercial use, distribution and reproduction in any medium, provided the work is properly cited.

* Address correspondence to this author at the Institute for Medical Informatics, Statistics and Epidemiology, University of Leipzig, Haertelstrasse 16-18 04107, Leipzig, Germany; Tel: +49 341 97 16121; Fax: +49 341 97 16109; E-mail: dirk.hasenclever@imise.uni-leipzig.de


Abstract

Measures of association play a role in selecting 2×2 tables exhibiting strong dependence in high-dimensional binary data. Several measures are in use differing on specific tables and in their dependence on the margins. We study a 2-dimensional group of margin transformations on the 3-dimensional manifold 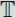of all 2×2 probability tables. The margin transformations allow introducing natural coordinates that identify 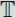with the real 3-space such that the x-axis corresponds to 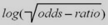and margins vary on planes x =const. We use these coordinates to visualise and compare measures of association with respect to their dependence on the margins given the odds-ratio, their limit behaviour when cells approach zero and their weighting properties. We propose a novel measure of association in which tables with single small entries are up-weighted but those with skewed margins are down-weighted according to the relative entropy among the tables of the same odds-ratio.

Keywords: Entropy, Margin, Measures of association, Odds-ratio, Statistical dependence, Two by two probability tables.